This topic is a quick review for readers who need a reminder about this area of mathematics. If you’re familiar with trigonometry, you can skip this topic. If you find this topic difficult to follow, you might consult a more basic reference on mathematics.
Trigonometric functions are principally used to model or describe:
The three basic trigonometric functions are derived from an angle rotating about a unit circle.
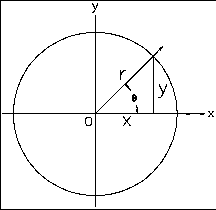
Trigonometric functions based on the unit circle
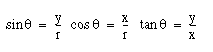
The tangent function is undefined for x=0. Another way to define the tangent is:
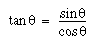
Because XYR defines a right-angled triangle, the relation between the sine and cosine is:

The graphs of the basic trigonometric functions illustrate their cyclical nature.

Graphs of basic trigonometric functions
The sine and cosine functions yield the same values, but the phase differs along the X axis by /2: in other words, 90 degrees.
The inverse functions for the trigonometric functions are the arc functions; the inverse only applies to values of x restricted by –/2 ≤ X ≤ /2. The graphs for these functions appear like the basic trigonometric function graphs, but turned on their sides.
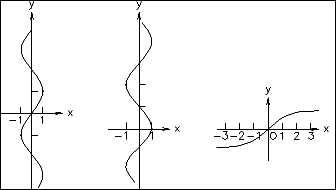
Graphs of basic arc functions
The hyperbolic functions are based on the exponential constant e instead of on circular measurement. However, they behave similarly to the trigonometric functions and are named for them. The basic hyperbolic functions are:

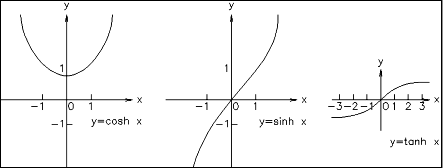
Graphs of basic hyperbolic functions